scipy.signal.

max_len_seq#

scipy.signal.max_len_seq(nbits, state=None, length=None, taps=None)[源代码]#

最大长度序列 (MLS) 生成器。

参数:

nbitsint: 要使用的位数。生成的序列的长度将为 (2**nbits) - 1。请注意，生成长序列（例如，大于 nbits == 16）可能需要很长时间。
statearray_like，可选: 如果为数组，则长度必须为 nbits，并且将被转换为二进制（布尔）表示。如果为 None，则将使用全 1 的种子，从而产生可重复的表示。如果 state 全部为零，则会引发错误，因为这是无效的。默认值：None。
lengthint，可选: 要计算的样本数。如果为 None，则计算整个长度 (2**nbits) - 1。
tapsarray_like，可选: 要使用的多项式抽头（例如，对于 8 位序列，[7, 6, 1]）。如果为 None，将自动选择抽头（对于高达 nbits == 32 的情况）。

返回:

seq数组: 生成的 0 和 1 的 MLS 序列。
state数组: 移位寄存器的最终状态。

备注

MLS 生成算法在以下位置进行了通用描述：

https://en.wikipedia.org/wiki/Maximum_length_sequence

抽头的默认值专门取自 nbits 的每个值在以下位置列出的第一个选项：

https://web.archive.org/web/20181001062252/http://www.newwaveinstruments.com/resources/articles/m_sequence_linear_feedback_shift_register_lfsr.htm

在版本 0.15.0 中添加。

示例

MLS 使用二进制约定

>>> from scipy.signal import max_len_seq
>>> max_len_seq(4)[0]
array([1, 1, 1, 1, 0, 1, 0, 1, 1, 0, 0, 1, 0, 0, 0], dtype=int8)

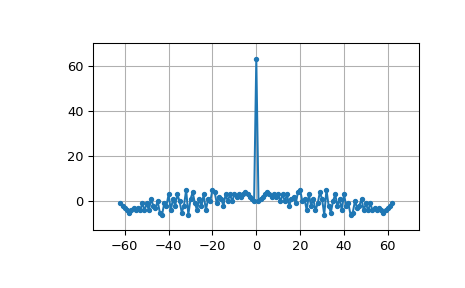
MLS 具有白色频谱（直流除外）

>>> import numpy as np
>>> import matplotlib.pyplot as plt
>>> from numpy.fft import fft, ifft, fftshift, fftfreq
>>> seq = max_len_seq(6)[0]*2-1  # +1 and -1
>>> spec = fft(seq)
>>> N = len(seq)
>>> plt.plot(fftshift(fftfreq(N)), fftshift(np.abs(spec)), '.-')
>>> plt.margins(0.1, 0.1)
>>> plt.grid(True)
>>> plt.show()

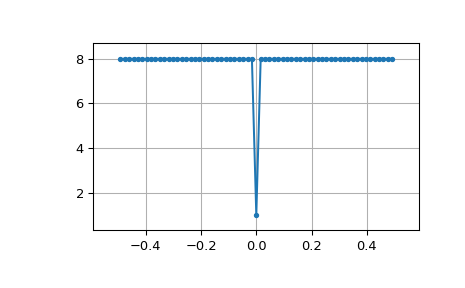
../../_images/scipy-signal-max_len_seq-1_00_00.png

MLS 的循环自相关是一个脉冲

>>> acorrcirc = ifft(spec * np.conj(spec)).real
>>> plt.figure()
>>> plt.plot(np.arange(-N/2+1, N/2+1), fftshift(acorrcirc), '.-')
>>> plt.margins(0.1, 0.1)
>>> plt.grid(True)
>>> plt.show()

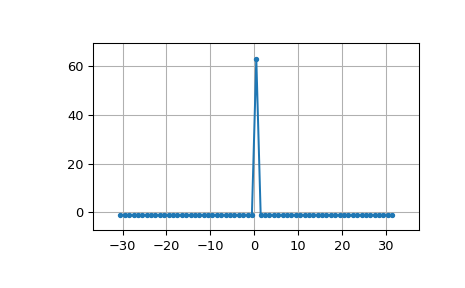
../../_images/scipy-signal-max_len_seq-1_01_00.png

MLS 的线性自相关近似于一个脉冲

>>> acorr = np.correlate(seq, seq, 'full')
>>> plt.figure()
>>> plt.plot(np.arange(-N+1, N), acorr, '.-')
>>> plt.margins(0.1, 0.1)
>>> plt.grid(True)
>>> plt.show()

../../_images/scipy-signal-max_len_seq-1_02_00.png